Introduction and Statement of the Fundamental Theorem of Calculus

Introduction

Introduction:

The fundamental theorem of calculus is a fundamental result in calculus that connects two concepts: differentiation and integration. It provides a powerful tool for solving problems involving rates of change, accumulation, and the evaluation of definite integrals.

Fundamental Theorem of Calculus:

The fundamental theorem of calculus has two parts: the first part relates differentiation and integration, while the second part relates definite integrals and antiderivatives.

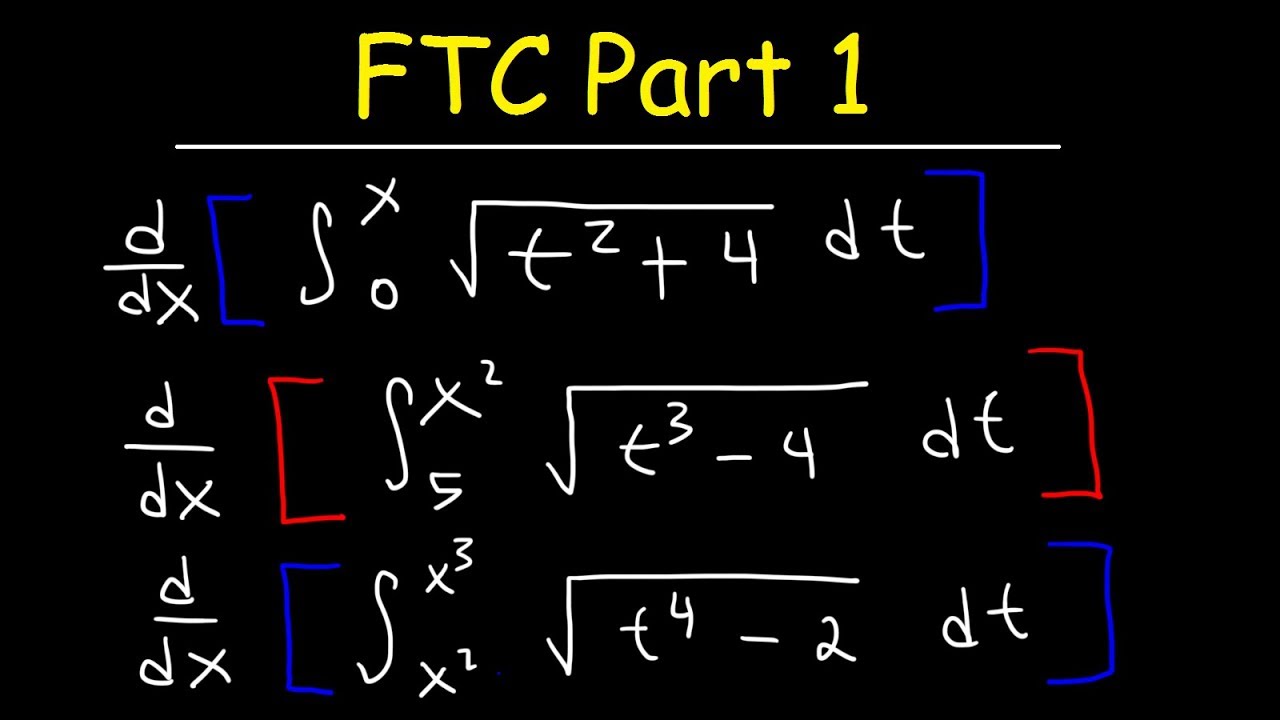
Part 1: Let f(x) be a continuous function on the interval [a, b], and let F(x) be an antiderivative of f(x) on the same interval. Then, the integral of f(x) from a to b can be evaluated by subtracting the value of F(x) at the lower limit (a) from the value of F(x) at the upper limit (b). Mathematically, this can be expressed as:

∫[a, b] f(x) dx = F(b) – F(a)

In simpler terms, this part of the theorem tells us that if we know an antiderivative of a function, we can find the definite integral of that function by evaluating the antiderivative at the limits of integration and subtracting those values.

Part 2: Suppose f(x) is a continuous function on an interval [a, b]. Let g(x) be any function that is continuous on [a, b] and differentiable on (a, b), with g'(x) = f(x) for all x in (a, b). Then, the definite integral of f(x) from a to b is equal to the difference between g(x) evaluated at the limits of integration:

∫[a, b] f(x) dx = g(b) – g(a)

This part of the theorem tells us that if we know the derivative of a function and we take an antiderivative of that derivative, it will give us the original function. Therefore, the definite integral of a derivative is equal to the difference of the function itself at the endpoints.

The fundamental theorem of calculus is a foundational concept in calculus and is widely used in various areas of mathematics, science, and engineering. It allows us to efficiently compute definite integrals by relating them to antiderivatives, enabling us to solve a range of problems involving rates of change and accumulation.

Statement of the Fundamental Theorem of Calculus

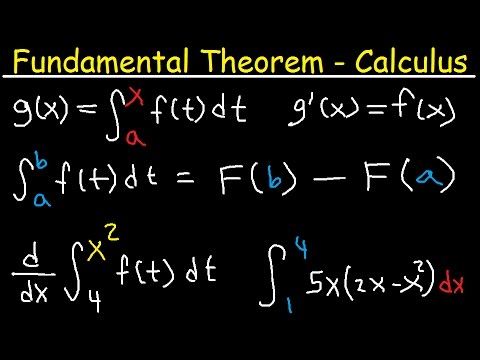
The Fundamental Theorem of Calculus states that if a function f is continuous on the interval [a, b] and F is an antiderivative of f on that interval, then the definite integral of f from a to b is equal to the difference in the values of F at the endpoints a and b. In other words, if F is an antiderivative of f, then:

∫[a to b] f(x) dx = F(b) – F(a)

This theorem establishes a connection between the concepts of differentiation and integration, allowing us to compute definite integrals by finding antiderivatives of the integrand function. It also provides a way to evaluate definite integrals without referencing Riemann sums, making it a fundamental tool in calculus.

Proof of the Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus states that if a function is continuous on a closed interval [a, b] and has an antiderivative F(x), then the definite integral of the function from a to b is equal to the difference between the antiderivative evaluated at the endpoints. In other words,

∫[a, b] f(x) dx = F(b) – F(a).

To prove this theorem, we can start by considering a function f(x) that is continuous on [a, b] and has an antiderivative F(x). Let’s define a new function G(x) as the definite integral of f(x) from a to x, so:

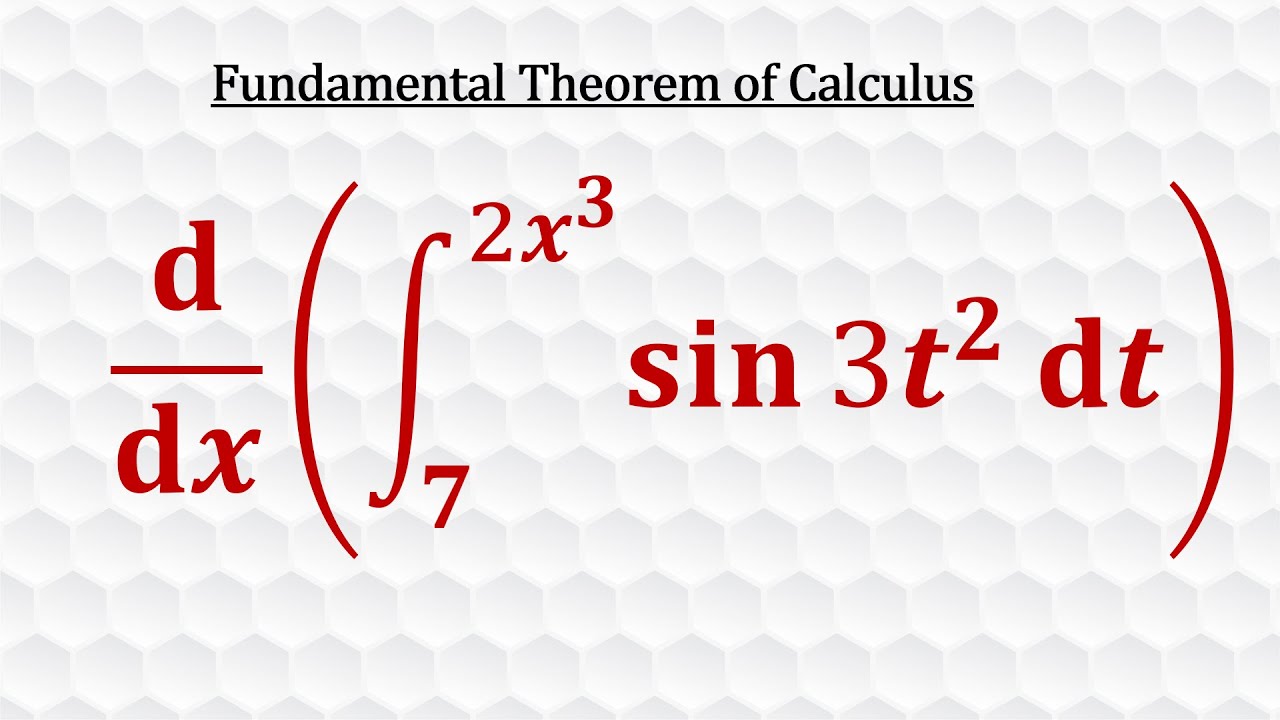
G(x) = ∫[a, x] f(t) dt.

Now, we need to show that G(x) is an antiderivative of f(x) on the interval [a, b]. To do this, we can differentiate G(x) using the First Fundamental Theorem of Calculus, which states that if a function is continuous on an interval [a, b] and has an antiderivative, then its derivative is the original function. So:

G'(x) = d/dx ∫[a, x] f(t) dt.

Using the Second Fundamental Theorem of Calculus, which states that if a function f(x) is continuous on an interval [a, b] and F(x) is an antiderivative of f(x) on that interval, then ∫[a, b] f(x) dx = F(b) – F(a), we can rewrite the derivative of G(x) as:

G'(x) = f(x).

Since G'(x) = f(x), we can conclude that G(x) is an antiderivative of f(x) on the interval [a, b].

Now, we can find the definite integral of f(x) from a to b by evaluating G(x) at the endpoints:

∫[a, b] f(x) dx = G(b) – G(a)

= ∫[a, b] f(t) dt – ∫[a, a] f(t) dt

= ∫[a, b] f(t) dt.

Since the integral of f(t) from a to a is zero, we have:

∫[a, b] f(x) dx = ∫[a, b] f(t) dt.

Therefore, we have proved the Fundamental Theorem of Calculus, which states that the definite integral of a function from a to b is equal to the difference between the antiderivative evaluated at the endpoints.

Applications of the Fundamental Theorem of Calculus

The Fundamental Theorem of Calculus has numerous practical applications in various fields, including physics, economics, and engineering. Here are a few notable applications:

1. Integrating Rates of Change: One application is in determining accumulated quantities or total change over a certain interval. For example, in physics, it can be used to calculate the displacement of an object from its velocity function, or to determine the total mass of a continuously changing distribution.

2. Area and Volume Calculation: The theorem can also be used to find the area between curves or the volume of three-dimensional shapes. By applying the definite integral, the Fundamental Theorem of Calculus can provide an efficient method for these calculations.

3. Solving Differential Equations: The Fundamental Theorem of Calculus enables the solution of initial value problems involving differential equations. By finding the antiderivative of a given function, it becomes easier to determine the function that satisfies a certain differential equation.

4. Optimization Problems: Calculus is often used to solve optimization problems, such as maximizing profit or minimizing cost, in economics or engineering. The Fundamental Theorem of Calculus plays a crucial role in this context by helping to find critical points and determine the optimal values.

5. Probability and Statistics: The theorem can be used in probability and statistics to calculate probabilities and expected values. By integrating probability density functions or probability mass functions, one can find the desired probabilities or expected values associated with random variables.

Overall, the applications of the Fundamental Theorem of Calculus are widespread and can be seen in various scientific and mathematical disciplines.

Conclusion

In conclusion, the Fundamental Theorem of Calculus is a fundamental result in calculus that establishes a connection between the concepts of differentiation and integration. It states that if a function is continuous on a closed interval and has an antiderivative, then the definite integral of the function over that interval can be evaluated by finding the difference between the antiderivative evaluated at the upper and lower bounds of the interval. This theorem is crucial in solving problems related to area, accumulation, and rates of change, and it forms the basis for many other concepts in calculus.

Topics related to Fundamental theorem of calculus