Definition of Definite Integral and Properties of Definite Integrals

Definition of Definite Integral

The definite integral is a mathematical concept used to calculate the accumulated value of a function over a specific interval. It is denoted by the symbol ∫ and consists of two components: the integrand, which is the function being integrated, and the limits of integration, which determine the interval over which the integration is performed.

To calculate the definite integral, one must evaluate the antiderivative (also known as the indefinite integral) of the integrand and then subtract the values of the antiderivative at the upper and lower limits of integration. This yields a numeric value that represents the accumulated area under the curve of the function within the given interval.

The definite integral is used in various fields of mathematics and science, such as in calculating areas, volumes, and determining the average value of a function. It is a fundamental concept in calculus and serves as a tool for solving a wide range of problems involving continuous functions.

Properties of Definite Integrals

The definite integral is a mathematical operation that allows us to calculate the area between a function and the x-axis over a given interval. It has several properties that make it a powerful tool in calculus:

1. Linearity: The definite integral is a linear operation, which means that the integral of the sum of two functions is equal to the sum of the integrals of the individual functions. Similarly, if you multiply a function by a constant, you can factor that constant out of the integral.

2. Change of Variables: The definite integral can be evaluated using a change of variables. This means that if we have a function f(x), and we substitute x = g(t) into the integral, we can rewrite the integral in terms of t and integrate with respect to t. This property is helpful in dealing with complicated functions.

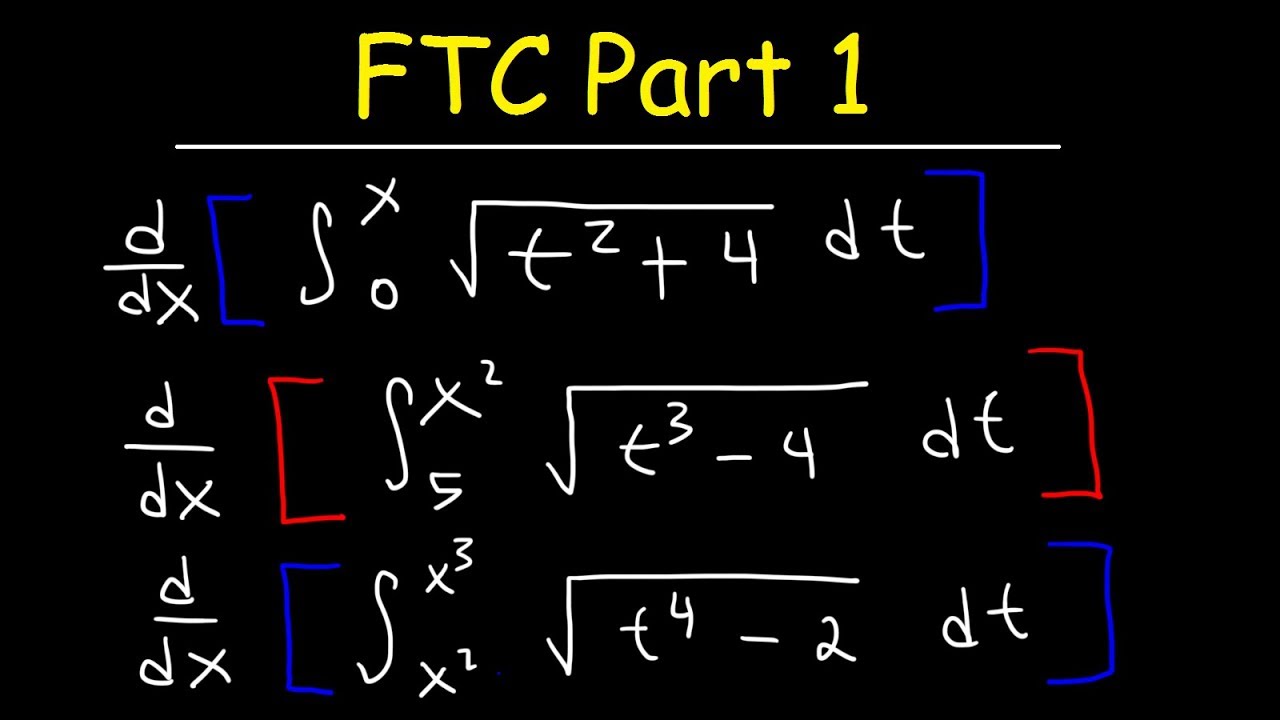
3. Fundamental Theorem of Calculus: The definite integral is closely related to the antiderivative of a function. The Fundamental Theorem of Calculus states that if F(x) is the antiderivative of f(x), then the definite integral of f(x) from a to b is equal to F(b) – F(a). This allows us to calculate definite integrals by finding antiderivatives.

4. Geometry Interpretation: The definite integral can be interpreted geometrically as the signed area between the graph of the function and the x-axis over the given interval. The area is positive if the function is above the x-axis and negative if the function is below the x-axis.

5. Approximation Methods: Definite integrals can be approximated using numerical methods such as Riemann sums or Simpson’s rule. These methods divide the interval into smaller subintervals and approximate the area using rectangles or polynomial approximations.

Overall, the definite integral is a powerful tool in calculus that allows us to calculate areas, find antiderivatives, and solve various problems in mathematics and science.

Techniques for Evaluating Definite Integrals

When evaluating definite integrals, there are several techniques that can be employed:

1. Direct Integration: This involves integrating the function directly using the power rule, product rule, quotient rule, or chain rule, depending on the form of the integrand.

2. Substitution: If the integrand involves a composition of functions, substitution can be used to simplify the integral. This usually involves letting u equal part of the integrand and substituting back to get the final answer.

3. Integration by Parts: This technique is useful when the integrand is a product of two functions. It involves using the formula: ∫uv dx = uv – ∫vu’ dx, where u and v’ are differentiable functions.

4. Partial Fraction Decomposition: This method is used when the integrand is a rational function. It involves decomposing the rational function into simpler fractions and integrating them individually.

5. Trigonometric Identities: When dealing with trigonometric functions, it can be beneficial to use trigonometric identities to simplify the integral. Common identities include sin^2(x) + cos^2(x) = 1 and 1 + tan^2(x) = sec^2(x).

6. Integration Tables: Some integrals have well-known results that can be looked up in integration tables or found online. These tables provide the solutions for common integrals.

7. Numerical Approximation: If an exact solution cannot be found or is too complicated, numerical methods such as the trapezoidal rule or Simpson’s rule can be used to approximate the value of the integral.

It is important to note that the choice of technique depends on the complexity of the integrand and the available tools. Sometimes, a combination of techniques may be required to evaluate a definite integral.

Applications of Definite Integrals

There are numerous applications of definite integrals in various fields such as physics, economics, geometry, and engineering. Some of the common applications of definite integrals include:

1. Finding areas: One of the primary applications of definite integrals is finding the area under curves or between curves. By taking the integral of a function over a given interval, we can calculate the area enclosed by the curve and the x-axis or between two curves.

2. Calculating volumes: Definite integrals can also be used to find the volume of three-dimensional shapes. By integrating the cross-sectional area of a shape along a given interval, we can determine the volume of the shape.

3. Computing probability: In statistics and probability theory, definite integrals are used to calculate the probability of an event occurring. Probability density functions (PDFs) are often integrated over a certain range to determine the likelihood of a specific outcome.

4. Evaluating work and energy: In physics, definite integrals are used to calculate work done by a force and the energy associated with a system. By integrating a force function with respect to displacement, we can determine the work done. Similarly, integrating a power function over time gives us the energy consumed or generated.

5. Solving differential equations: Definite integrals are used to solve differential equations and initial value problems. By integrating both sides of a differential equation, we can find a general solution to the equation and apply initial conditions to obtain a specific solution.

6. Modeling population growth: The concept of definite integrals is employed in mathematical modeling of population growth. By integrating the rate of change of population over time, we can determine the total population at any given time.

7. Finding centers of mass: Definite integrals can be used to find the coordinates of the center of mass for irregularly shaped objects. By integrating appropriate functions that represent mass distribution along different axes, we can determine the center of mass.

These are just a few examples of the many applications of definite integrals. They have a wide range of uses in various fields, allowing us to understand and analyze real-world phenomena more deeply.

Importance and Significance of Definite Integrals in Mathematics

The definite integral is a fundamental concept in mathematics that has importance and significance in a variety of areas. Here are some key points about the importance and significance of definite integrals:

1. Area under a curve: One of the most important applications of definite integrals is calculating the area under a curve. By integrating the function over a specific interval, we can find the exact area between the curve and the x-axis. This concept is widely used in calculus, physics, and engineering to solve real-world problems involving areas and volumes.

2. Accumulated change: Definite integrals also allow us to measure the accumulated change in a quantity over a given interval. For example, if we have a graph representing the velocity of an object over time, integrating the function from an initial time to a final time gives us the total displacement of the object during that time period. This idea is crucial in studying motion, economics, population growth, and various other fields.

3. Calculating averages: The definite integral plays a key role in calculating averages of various quantities. For example, the average value of a function over an interval can be found by dividing the definite integral of the function over that interval by the length of the interval. This concept is important in statistics and probability theory to determine average values, expected values, and probabilities.

4. Solving differential equations: Definite integrals are closely connected to solving differential equations. Many differential equations involve the derivative of an unknown function, and to find the original function, we need to use definite integrals. By integrating both sides of a differential equation, we can obtain a solution that satisfies the equation.

5. Applications in physics and engineering: Definite integrals are extensively used in physics and engineering to model and solve problems related to motion, work, energy, and electrical circuits. They help to analyze and compute quantities such as work done by a force, power, center of mass, moment of inertia, and many other physical and engineering phenomena.

Overall, the definite integral is a crucial tool in mathematics and has significant applications in various fields. It enables us to calculate areas, accumulate changes, determine averages, solve differential equations, and model real-world situations. Its versatility and wide range of applications make it an essential concept for understanding and solving a variety of mathematical and scientific problems.

Topics related to Definite integral