Definition of Complex Conjugation and Properties of Complex Conjugation

Definition of Complex Conjugation

Complex conjugation is an operation performed on a complex number in order to obtain its conjugate. In mathematical terms, if a complex number is expressed as a + bi, where a and b are real numbers and i is the imaginary unit, then its complex conjugate is given by a – bi. In other words, complex conjugation involves changing the sign of the imaginary part of the complex number while keeping the sign of the real part the same. This operation is denoted by adding a bar or asterisk above the complex number, for example, if z = a + bi, then its complex conjugate is represented as (bar{z}) or (z^*). Complex conjugation plays an important role in various mathematical and physical applications, such as in finding the absolute value of a complex number or in solving equations involving complex conjugates.

Properties of Complex Conjugation

Complex conjugation is an operation performed on a complex number, which involves changing the sign of its imaginary part while keeping its real part unchanged. It is denoted by putting a bar over the complex number, for example, if z is a complex number, then its complex conjugate is denoted by z̅.

Some properties of complex conjugation are:

1. Commutativity: For any complex number z, the complex conjugate of its complex conjugate is the original number. In symbolic terms, (z̅)̅ = z.

2. Linearity: Complex conjugation follows the rules of linearity. This means that for any complex numbers z1 and z2, and any scalar c, the complex conjugate of the sum of two complex numbers, or the product of a complex number and a scalar, is the same as the sum or product of their complex conjugates. Symbolically, (z1 + z2)̅ = z1̅ + z2̅ and (cz)̅ = c(z̅).

3. Conjugate of a real number: If a complex number z is purely real, meaning its imaginary part is zero, then its complex conjugate is simply itself. In other words, if z = a + 0i, where a is a real number, then z̅ = z.

4. Conjugate of a purely imaginary number: If a complex number z is purely imaginary, meaning its real part is zero, then its complex conjugate is the negative of itself. In other words, if z = 0 + bi, where b is a real number and i is the imaginary unit, then z̅ = -z.

5. Multiplication property: The complex conjugate of the product of two complex numbers is equal to the product of their complex conjugates. Symbolically, (zw)̅ = z̅w̅.

These properties of complex conjugation are commonly used in various areas of mathematics and engineering, such as complex analysis, signal processing, and quantum mechanics.

Applications of Complex Conjugation

Complex conjugation is a mathematical operation that involves changing the sign of the imaginary part of a complex number. It plays a significant role in various mathematical and scientific applications. Here are some common applications of complex conjugation:

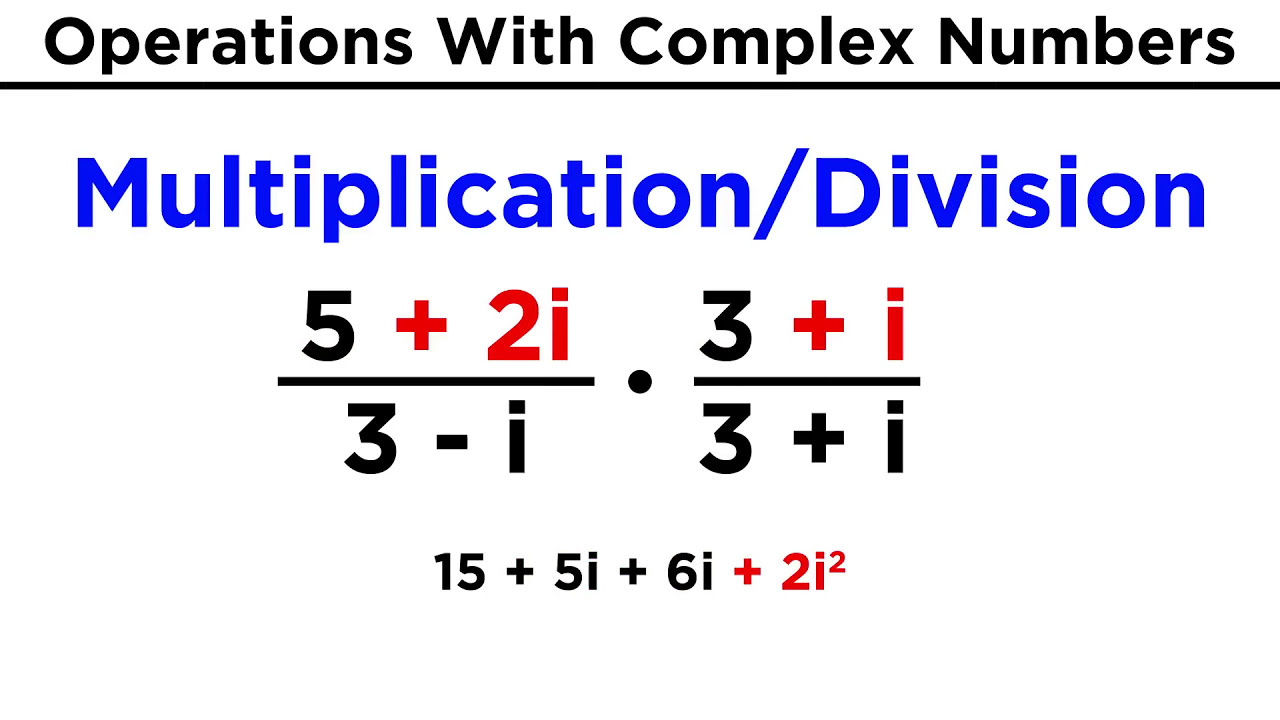
1. Complex Arithmetic: Complex conjugation is used when performing arithmetic operations on complex numbers. For example, when dividing one complex number by another, it is customary to multiply both the numerator and denominator by the conjugate of the denominator. This technique helps simplify the calculations and obtain real-valued results.

2. Complex Functions and Analysis: In complex analysis, functions that satisfy certain conditions are said to be analytically continued. Complex conjugation is often used to define new functions by reflecting, rotating, or mirroring existing ones. This allows mathematicians to extend the domain of a function and explore its behavior in different regions of the complex plane.

3. Signal Processing: In signal processing, the complex conjugate of a signal is commonly used for various purposes. For example, in the field of digital communication, the complex conjugate is applied to a transmitted signal to account for phase shifts and fading effects caused by the propagation medium. This operation helps recover the original signal at the receiving end.

4. Physics and Engineering: Complex conjugation is also relevant in physics and engineering fields. In electromagnetic theory, complex conjugation is used to describe the reflection of electromagnetic waves from surfaces. It helps analyze the behavior of these waves when interacting with various materials or boundary conditions. Additionally, in quantum mechanics, complex conjugation is used to calculate probabilities and expectation values in wave function calculations.

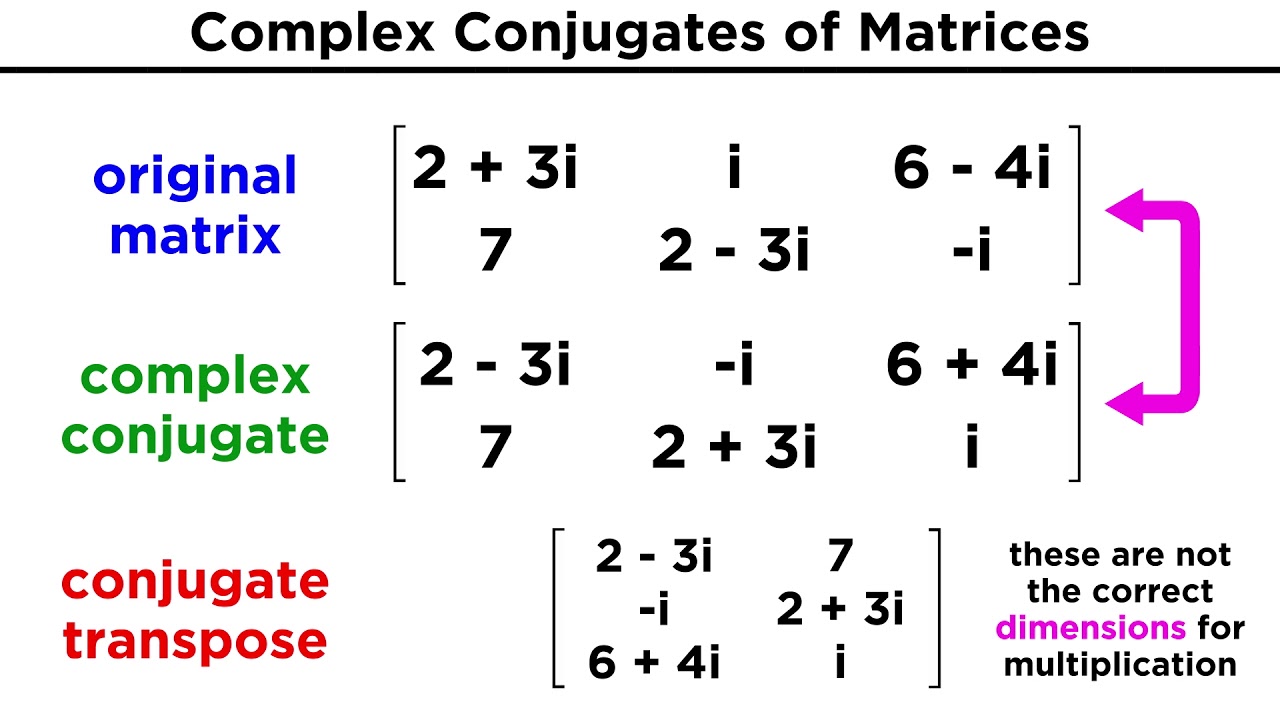
5. Complex Conjugate Pairs: Complex conjugate pairs, which consist of a complex number and its conjugate, have several applications in different areas. In linear algebra, complex conjugate pairs are essential when dealing with matrices and vectors. They help define and manipulate Hermitian matrices, unitary matrices, and orthogonal vectors. Moreover, in the study of equations, roots of a polynomial often come in complex conjugate pairs, which are useful in finding solutions to equations.

6. Conjugate Gradient Method: The conjugate gradient method is an iterative algorithm used to solve systems of linear equations, particularly in numerical analysis and scientific simulations. This method relies on complex conjugation to find a solution efficiently while minimizing computational effort.

7. Fourier Analysis: Complex conjugation is widely used in Fourier analysis, a mathematical technique that decomposes a function into a sum of sinusoidal components. The complex conjugate of a signal plays a crucial role in the calculation of Fourier transforms, signal synthesis, and identifying symmetry properties.

These are just a few examples of the numerous applications of complex conjugation. Its versatility and utility make it an indispensable tool in mathematical modeling, signal processing, and various scientific disciplines.

Complex Conjugate Roots

When solving quadratic equations, it is possible for the roots to be complex numbers. Complex conjugate roots occur when the discriminant of the quadratic equation is negative.

Complex conjugate roots come in pairs, and they have the form a + bi and a – bi, where a and b are real numbers and i is the imaginary unit (√-1).

Complex conjugation is the process of changing the sign of the imaginary part of a complex number. It involves taking the complex number a + bi and replacing bi with -bi, resulting in a – bi. Complex conjugation is denoted by a bar on top of the complex number, such as z̄ (read as “z bar”).

Complex conjugation has several properties:

1. The complex conjugate of a sum is equal to the sum of the complex conjugates: (a + bi) + (c + di) = a + bi + c + di = (a + c) + (b + d)i.

2. The complex conjugate of a product is equal to the product of the complex conjugates: (a + bi)(c + di) = (ac + adi + bci + bdi^2) = (ac – bd) + (ad + bc)i.

3. The complex conjugate of a complex conjugate is equal to the original complex number: (a + bi)̄ = a – bi.

Complex conjugate roots play an essential role in various mathematical areas, including complex analysis, signal processing, and electrical engineering. They have significant applications in solving equations, understanding waveforms, and analyzing the behavior of systems involving complex numbers.

Complex Conjugate Symmetry

Complex conjugate symmetry, also known as complex conjugation, is a property of complex numbers.

A complex number is expressed as a sum of a real part and an imaginary part, written as a + bi, where a and b are real numbers and i is the imaginary unit (√(-1)). The complex conjugate of a complex number a + bi is obtained by changing the sign of the imaginary part, resulting in a – bi.

The complex conjugate symmetry states that if a complex number z is given, then its complex conjugate, denoted as z*, is also a valid complex number. In other words, if z = a + bi, then z* = a – bi.

This symmetry property has some important implications in complex analysis and other areas of mathematics. For example, the product of a complex number and its complex conjugate is always a real number. In other words, if z = a + bi, then z*z* = (a + bi)(a – bi) = a^2 + b^2, which is a real number.

Complex conjugate symmetry is also used in finding the roots of complex polynomials. If a polynomial equation has a complex root, then its complex conjugate is also a root. This property helps in simplifying and solving polynomial equations involving complex numbers.

Overall, complex conjugate symmetry is a key property that allows for various operations on complex numbers and plays a crucial role in mathematical analysis and applications.

Topics related to Complex conjugation