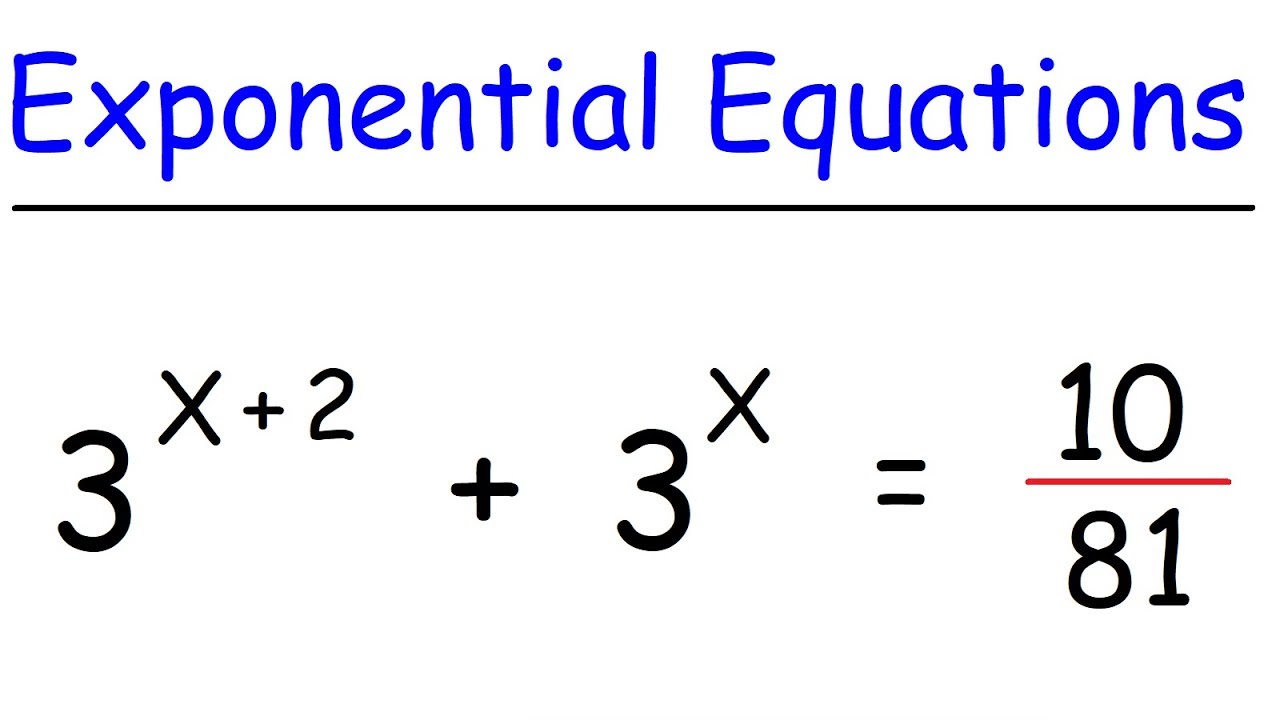Definition of exponential function and Properties of exponential functions

Definition of exponential function

An exponential function is a mathematical function of the form f(x) = a^x, where “a” is a constant greater than zero, and “x” is the exponent. This function is characterized by the fact that the value of the function increases or decreases at a faster rate as the input value increases. In other words, the function exhibits exponential growth or decay. The constant “a” is known as the base of the exponential function and determines the rate of growth or decay. Exponential functions have various applications in fields such as finance, biology, physics, and computer science.

Properties of exponential functions

The properties of exponential functions refer to the unique characteristics and behavior of functions that have a base raised to a variable exponent. Here are some key properties:

1. Exponential growth: Exponential functions with a positive base (greater than 1) exhibit exponential growth. As the variable increases, the function grows at an increasing rate. The graph of such an exponential function is always an upward curve.

2. Exponential decay: Exponential functions with a base between 0 and 1 (exclusive) exhibit exponential decay. As the variable increases, the function decreases at an increasing rate. The graph of such an exponential function is always a downward curve.

3. Asymptote: Exponential functions never cross or touch the x-axis. Instead, they approach it or move away from it, depending on whether it represents exponential growth or decay. The x-axis acts as a horizontal asymptote.

4. Initial value: The initial value or initial condition of an exponential function is the value of the function when the independent variable is zero. It often represents the starting point or initial amount in applications.

5. Growth/decay factor: The base of the exponential function determines the growth or decay factor. For growth functions, the growth factor is greater than 1, while for decay functions, the decay factor is between 0 and 1.

6. Exponential properties: Exponential functions have certain algebraic properties. For example, when multiplying exponential functions with the same base, the exponents can be added together. Similarly, when dividing exponential functions with the same base, the exponents can be subtracted.

7. Half-life: Exponential decay functions are often used to model processes that undergo radioactive decay or other natural decay phenomena. The half-life refers to the time it takes for the initial value to decrease by half. It can be calculated using the formula t = ln(0.5) / k, where t is the half-life and k is the decay constant.

These properties make exponential functions useful in various fields, such as finance, biology, physics, and population studies, where exponential growth and decay occur.

Common applications of exponential functions

Exponential functions are applied to various fields and have numerous practical applications. Some common applications of exponential functions include:

1. Finance: Exponential growth and decay functions are used to model compound interest, investments, and depreciation of assets over time.

2. Population Growth: Exponential functions are used to study population growth or decline over time. They can help project future population sizes and analyze birth and mortality rates.

3. Radioactive Decay: Exponential functions are used to model the decay of radioactive substances. The rate at which radioactive isotopes decay can be described by exponential decay functions.

4. Biology and Medicine: Exponential growth functions are used to model the growth of bacteria, viruses, and other organisms. They can also be applied to study the spread of diseases and the effectiveness of vaccines.

5. Physics: Exponential functions are used to describe phenomena such as radioactive decay, wave interference, and decay of electrical current in circuits.

6. Computer Science: Exponential functions are used in computer science to analyze algorithms, study exponential time complexity, and model the growth of data storage capacities over time.

7. Economics: Exponential growth functions are used in economics to model economic growth, inflation rates, and the spread of economic indicators like GDP.

8. Environmental Science: Exponential functions are used to model the growth of environmental pollution, deforestation rates, and climate change effects.

9. Marketing and Advertising: Exponential functions are used in marketing to model the growth of customer base, sales, and market share over time.

10. Epidemiology: Exponential functions are used to analyze the spread of infectious diseases, predict outbreaks, and evaluate the impact of interventions like social distancing or vaccination campaigns.

These are just a few examples of how exponential functions are applied in various fields. Their ability to describe rapid growth or decay processes makes them valuable tools for analyzing and predicting changes in many real-world situations.

Graphing exponential functions

An exponential function is a function of the form f(x) = a^x, where a is a positive constant known as the base and x is the exponent.

When graphing exponential functions, the shape of the graph depends on the value of the base a. If a is greater than 1, the graph will increase as x increases, creating a curve that starts at the origin (0,1) and moves upward to the right. This is known as exponential growth.

On the other hand, if a is between 0 and 1, the graph will decrease as x increases, creating a curve that starts at the origin and moves downward to the right. This is known as exponential decay.

Exponential functions also have some important properties:

1. The graph of f(x) = a^x will always pass through the point (0,1), regardless of the value of a.

2. The function is always positive, meaning that the graph will only appear in the quadrant where y > 0.

3. As x approaches negative infinity, the value of the function will approach 0. As x approaches positive infinity, the value of the function will either approach infinity (for exponential growth) or approach 0 (for exponential decay).

To graph an exponential function, you can choose some x-values and evaluate the corresponding y-values using the function equation. You can then plot these points on the coordinate plane and connect them with a smooth curve.

Remember to consider the domain and range of the exponential function when graphing. The domain is the set of all real numbers, while the range depends on the base: for exponential growth, the range is y > 0, and for exponential decay, the range is 0 < y < 1.

Overall, graphing exponential functions involves understanding the behavior of the base and using this knowledge to determine the shape, direction, and key points of the graph.

Examples of exponential functions

Here are some examples of exponential functions:

1. Population Growth: The function P(t) = P₀ * e^(kt), where P₀ is the initial population, k is the growth rate, and t is time, represents the exponential growth of a population over time.

2. Compound Interest: The formula A(t) = P₀ * (1 + r/n)^(nt), where A(t) is the amount after time t, P₀ is the principal amount, r is the annual interest rate, n is the number of times the interest is compounded per year, and t is time, represents the exponential growth of money with compound interest.

3. Radioactive Decay: The equation N(t) = N₀ * e^(-λt), where N₀ is the initial quantity of a radioactive substance, λ is the decay constant, and t is time, represents the exponential decay of a radioactive substance over time.

4. Carbon-14 Dating: The equation M(t) = M₀ * e^(-kt), where M(t) is the remaining mass of carbon-14, M₀ is the initial mass of carbon-14, k is the rate of decay of carbon-14, and t is time, represents the exponential decay of carbon-14 in a sample, which is useful for determining the age of organic materials.

5. Sound Intensity: The formula I(x) = I₀ / (x²), where I(x) is the sound intensity at a distance x from the source, I₀ is the initial sound intensity, and x is the distance from the source, represents the exponential decay of sound intensity with distance.

These are just a few examples of exponential functions, which describe phenomena that grow or decay at an increasing rate over time or distance.

Topics related to Exponential function