Definition of indefinite integral and Properties and rules of indefinite integrals

Definition of indefinite integral

The indefinite integral, also known as the antiderivative, is a mathematical concept in calculus. It is used to find a function that, when differentiated, produces a given function.

In simpler terms, the indefinite integral represents a whole family of functions whose derivative is equal to the original function. It is denoted by the ∫ symbol, followed by the function to be integrated, and is typically followed by the variable of integration.

Mathematically, if we have a function f(x), then its indefinite integral F(x) is expressed as:

∫ f(x) dx = F(x) + C

where F(x) is a function whose derivative is f(x), dx denotes the variable of integration (usually x), and C is the constant of integration.

The constant of integration, represented by C, is added because the derivative of a constant is zero. Since the indefinite integral represents a whole family of functions, each differing by a constant, the constant of integration is necessary to account for this variability.

Finding the indefinite integral primarily involves reversing the process of differentiation. A variety of integration techniques and rules, such as substitution, integration by parts, and trigonometric identities, are utilized to compute the indefinite integral for various functions.

Properties and rules of indefinite integrals

The indefinite integral, also known as antiderivative, is a mathematical operation that can be used to find a function whose derivative is the original function. It is denoted by the symbol ∫.

There are several properties and rules associated with indefinite integrals:

1. Linearity: The indefinite integral is a linear operation, which means that the integral of the sum of two functions is equal to the sum of their integrals. In mathematical notation, this property can be expressed as:

∫(f(x) + g(x)) dx = ∫f(x) dx + ∫g(x) dx

2. Constant multiple rule: If a constant multiple is multiplied by a function, the indefinite integral of the product is equal to the constant multiple multiplied by the indefinite integral of the original function:

∫k * f(x) dx = k * ∫f(x) dx

3. Power rule: If the function to be integrated is of the form x^n, where n is any real number except -1, the indefinite integral is given by:

∫x^n dx = (x^(n+1))/(n+1) + C

4. Constant of integration: When integrating a function, we always add a constant term at the end of the result. This constant is known as the constant of integration, denoted by C. This is because the derivative of a constant is always zero.

5. Substitution rule: The substitution rule is a powerful technique used to simplify integrals. It allows for a change of variables to make the integral more manageable. The basic idea is to substitute a new variable in place of the original variable in the integrand and then perform the integration with respect to the new variable.

6. Integration by parts: Integration by parts is another technique used to simplify integrals. It is derived from the product rule of differentiation and allows for the integration of the product of two functions.

7. Trigonometric integrals: There are several specific rules for integrating trigonometric functions such as sin(x), cos(x), sec(x), etc. These rules involve trigonometric identities and can be used to simplify and solve integrals involving trigonometric functions.

It is important to note that the indefinite integral does not give a specific value as an answer, but rather gives a family of functions with varying constant terms. To find a particular value or definite integral, bounds of integration must be provided.

Techniques for solving indefinite integrals

There are several techniques that can be used to solve indefinite integrals. Here are some commonly used methods:

1. Power Rule: If the integrand is a power of x, you can use the power rule to find the indefinite integral. For example, the integral of x^n with respect to x is (x^(n+1))/(n+1), where n is any real number except -1.

2. Trigonometric Substitution: This technique is used when the integrand involves trigonometric functions. By substituting a trigonometric expression for a given variable, you can simplify the integral. This method is particularly useful for integrals involving square roots and certain trigonometric identities.

3. Integration by Parts: This method is based on the product rule of differentiation. It is useful for integrating the product of two functions. The formula for integration by parts is ∫u dv = uv – ∫v du, where u and v represent functions of x.

4. Partial Fraction Decomposition: This method is used to integrate rational functions, which are fractions of polynomials. By decomposing the fraction into simpler fractions with known integrals, you can simplify the integration process.

5. Substitution: This technique involves substituting a new variable or expression for the original variable in the integrand. This can help simplify the integral by reducing it to a known form. Common substitutions include u-substitution, trigonometric substitution, and exponential substitution.

6. Special Functions: Some integrals can be expressed in terms of special functions, such as exponential functions, logarithmic functions, or hyperbolic functions. These special functions have well-defined integrals, and their properties can be used to solve various types of integrals.

It’s important to note that not all integrals can be solved using these techniques alone. Some integrals require more advanced methods or may not have a closed-form solution.

Applications of indefinite integrals

The indefinite integral, also known as antiderivative, is a fundamental concept in calculus. It is the reverse process of differentiation and has several applications in various fields.

1. Calculating displacement: The indefinite integral can be used to determine the position or displacement of an object over time. By integrating the velocity function with respect to time, we can find the position function.

2. Finding areas: The indefinite integral can be used to calculate the area under a curve. This is helpful in many areas, such as calculating the area of a region, determining the total sales or profit over a specific time period, or finding the area between two curves.

3. Solving differential equations: Differential equations describe the relationship between a function and its derivatives. The indefinite integral is often used to solve these equations by finding the antiderivative of the given function.

4. Evaluating definite integrals: The indefinite integral is a tool used to evaluate definite integrals. By finding the antiderivative of a function and using the Fundamental Theorem of Calculus, we can calculate the exact value of a definite integral.

5. Probability and statistics: The indefinite integral is used in probability and statistics to calculate probabilities, expected values, and cumulative distribution functions for continuous random variables.

6. Physics and engineering: In physics and engineering, the indefinite integral is commonly used to determine quantities such as work, energy, power, and electric or magnetic fields. It helps in understanding the relationship between these quantities and their rates of change.

7. Economics and finance: The indefinite integral is used to analyze economic and financial concepts such as marginal cost, marginal revenue, consumer surplus, producer surplus, and present value. It helps in understanding the relationship between these variables and their rates of change.

Overall, the indefinite integral has wide-ranging applications in many fields, enabling us to solve problems involving rates of change, accumulation, and quantities related to area or volume. It is a powerful tool in mathematics and its applications are vast and diverse.

Examples of indefinite integrals

Here are some examples of indefinite integrals:

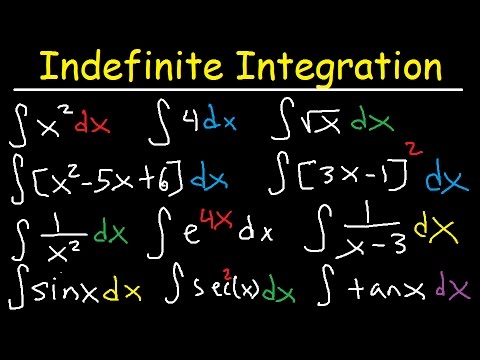
1. ∫ x^2 dx = (1/3) x^3 + C

2. ∫ e^x dx = e^x + C

3. ∫ 3x^2 + 2x – 1 dx = x^3 + x^2 – x + C

4. ∫ sin(x) dx = -cos(x) + C

5. ∫ 1/x dx = ln|x| + C

6. ∫ 2cos(2x) dx = sin(2x) + C

7. ∫ √x dx = (2/3) x^(3/2) + C

8. ∫ 1/(x^2 + 1) dx = arctan(x) + C

9. ∫ 3e^(-2x) dx = -(3/2) e^(-2x) + C

10. ∫ x^3 + 2x^2 – 5x + 6 dx = (1/4) x^4 + (2/3) x^3 – (5/2) x^2 + 6x + C

Note: In all these examples, C represents the constant of integration.

Topics related to Indefinite integral