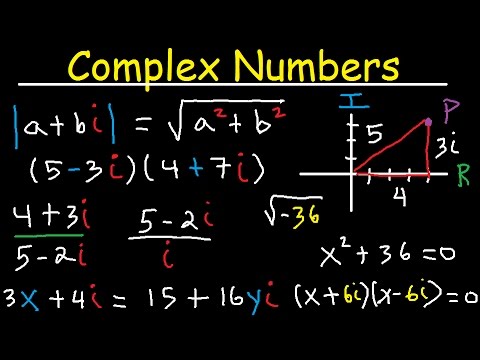
Definition of the Complex Plane and Components of the Complex Plane

Definition of the Complex Plane

The complex plane, also known as the Argand plane or the Gauss plane, is a mathematical concept that represents complex numbers visually. It consists of a two-dimensional coordinate system where the horizontal axis represents the real numbers and the vertical axis represents the imaginary numbers.

In the complex plane, each point corresponds to a unique complex number, which can be written in the form a + bi, where a is the real part and b is the imaginary part. The point (a, b) on the complex plane corresponds to the complex number a + bi.

The complex plane allows for geometric representations and operations on complex numbers. The distance from the origin (0,0) to a point on the complex plane represents the magnitude or modulus of the corresponding complex number. The angle between the positive real axis and the line connecting the origin and the point represents the argument or phase of the complex number.

The complex plane is a useful tool in many areas of mathematics, including complex analysis, electrical engineering, and physics. It provides a visual way to understand and manipulate complex numbers, making them more tangible and easier to work with.

Components of the Complex Plane

The complex plane is a two-dimensional space where each point corresponds to a complex number. It is formed by combining the real number line and the imaginary number line. The components of the complex plane are:

1. Real Axis: This is the horizontal axis that represents the real numbers. It is equivalent to the x-axis on a Cartesian coordinate system.

2. Imaginary Axis: This is the vertical axis that represents the imaginary numbers. It is equivalent to the y-axis on a Cartesian coordinate system.

3. Origin: The point where the real axis and imaginary axis intersect is called the origin. It represents the number 0 in the complex plane.

4. Complex Numbers: Each point in the complex plane corresponds to a unique complex number. Complex numbers are represented in the form a + bi, where “a” is the real part and “b” is the imaginary part.

5. Quadrants: The complex plane is divided into four quadrants based on the signs of the real and imaginary parts. These quadrants, named as the first, second, third, and fourth quadrants, are similar to the quadrants in a Cartesian coordinate system.

The complex plane is a useful tool for visualizing complex numbers and performing operations on them, such as addition, subtraction, multiplication, and division. It allows for geometric interpretations of complex numbers and helps in understanding their relationships.

Operations on the Complex Plane

The complex plane, also known as the Argand plane or the complex plane, is a geometric representation of complex numbers. It consists of a set of coordinate axes, with the real numbers represented on the horizontal axis and the imaginary numbers represented on the vertical axis.

Operations on the complex plane involve manipulating complex numbers using arithmetic operations such as addition, subtraction, multiplication, and division.

Addition: To add two complex numbers, we simply add their real parts and their imaginary parts separately. For example, if we have two complex numbers z1 = a + bi and z2 = c + di, then their sum is z1 + z2 = (a + c) + (b + d)i.

Subtraction: To subtract two complex numbers, we subtract their real parts and imaginary parts separately. For example, z1 – z2 = (a – c) + (b – d)i.

Multiplication: To multiply two complex numbers, we use the distributive property and FOIL method. For example, if we have two complex numbers z1 = a + bi and z2 = c + di, then their product is z1 * z2 = (a*c – b*d) + (a*d + b*c)i.

Division: To divide two complex numbers, we multiply both the numerator and denominator by the complex conjugate of the denominator. The complex conjugate of a complex number a + bi is given by a – bi. For example, if we have two complex numbers z1 = a + bi and z2 = c + di, then their division is z1 / z2 = (ac + bd)/(c^2 + d^2) + ((bc – ad)/(c^2 + d^2))i.

These operations can be visualized on the complex plane by representing each complex number as a point in the plane. Addition corresponds to vector addition, multiplication corresponds to a combination of scaling and rotation, and division corresponds to a combination of scaling, rotation, and inversion.

The complex plane is a powerful tool in mathematics and physics, allowing us to visualize and analyze complex numbers and their relationships. It is used in various fields, including electrical engineering, control systems, signal processing, and quantum mechanics.

Applications of the Complex Plane in Mathematics

The complex plane, also known as the Argand plane, is a useful tool in mathematics for studying complex numbers and their properties. Here are some applications of the complex plane:

1. Representation of complex numbers: In the complex plane, a complex number z = a + bi is represented as a point with coordinates (a, b). This visual representation helps in understanding the properties of complex numbers and their interactions.

2. Geometric interpretation: The complex plane allows us to interpret complex numbers geometrically. In this interpretation, the real part corresponds to the x-coordinate and the imaginary part corresponds to the y-coordinate. The magnitude of a complex number is related to its distance from the origin, and the argument (or angle) of a complex number is related to its position with respect to the x-axis.

3. Visualization of operations: In the complex plane, arithmetic operations on complex numbers such as addition, subtraction, multiplication, and division have geometric interpretations. For example, addition of two complex numbers corresponds to vector addition, and multiplication corresponds to scaling and rotation.

4. Polar form and De Moivre’s theorem: The complex plane aids in the understanding of polar form, where complex numbers are represented by their magnitude and argument. De Moivre’s theorem, which deals with raising complex numbers to a power, is also visualized easily in the complex plane.

5. Root-finding: The complex plane can be used to find the roots of complex polynomials. The roots correspond to the points where the polynomial intersects the x-axis in the complex plane.

6. Analytic functions: The complex plane is crucial for the study of analytic functions, which are complex-valued functions that possess certain properties. The properties of analytic functions can be understood and visualized using the complex plane, such as the concept of holomorphicity and the Cauchy-Riemann equations.

7. Residue theory: Residue theory, a powerful tool in complex analysis, involves calculating the residues of complex functions at singularities. The complex plane is used to locate and analyze these singularities, which are characterized by poles or branch points.

8. Conformal mapping: Conformal mapping is a technique that maps one region of the complex plane to another, preserving the angles between intersecting curves. It has applications in fluid dynamics, electrical engineering, and geometry, to name a few. The complex plane provides a visual representation of these mappings.

The complex plane is a versatile tool that bridges algebra, geometry, and complex analysis, making it indispensable in various branches of mathematics and its applications.

Conclusion and Significance of the Complex Plane in Mathematics

In conclusion, the complex plane is a fundamental concept in mathematics that extends the real number line into a two-dimensional space. It provides a geometric representation of complex numbers, which are numbers of the form a + bi, where a and b are real numbers and i is the imaginary unit.

The complex plane is highly significant in various branches of mathematics. It allows for a better understanding and visualization of complex numbers, which play a crucial role in many areas of mathematics, including algebra, analysis, and geometry.

One of the significant features of the complex plane is its ability to represent operations involving complex numbers in a geometric manner. Addition and subtraction of complex numbers correspond to vector addition and subtraction on the complex plane. Multiplication and division of complex numbers can be represented using rotation and scaling operations on the plane.

Furthermore, the complex plane provides a powerful tool for solving polynomial equations. The Fundamental Theorem of Algebra states that every polynomial equation with complex coefficients has at least one complex root. Using the complex plane, complex roots can be represented as points on the plane, making it easier to determine the number and location of roots.

The complex plane also allows for the representation and analysis of periodic functions, such as exponential and trigonometric functions. The relationship between complex numbers and trigonometry is particularly notable, as complex numbers can be expressed using Euler’s formula, e^(ix) = cos(x) + i*sin(x), where e is the base of the natural logarithm and i is the imaginary unit.

In summary, the complex plane is a powerful tool in mathematics that provides a geometric representation of complex numbers. It enhances our understanding of complex numbers and allows for the visualization and analysis of various mathematical concepts and operations. Its significance extends to many areas of mathematics, making it a crucial tool for mathematicians and scientists alike.

Topics related to Complex plane