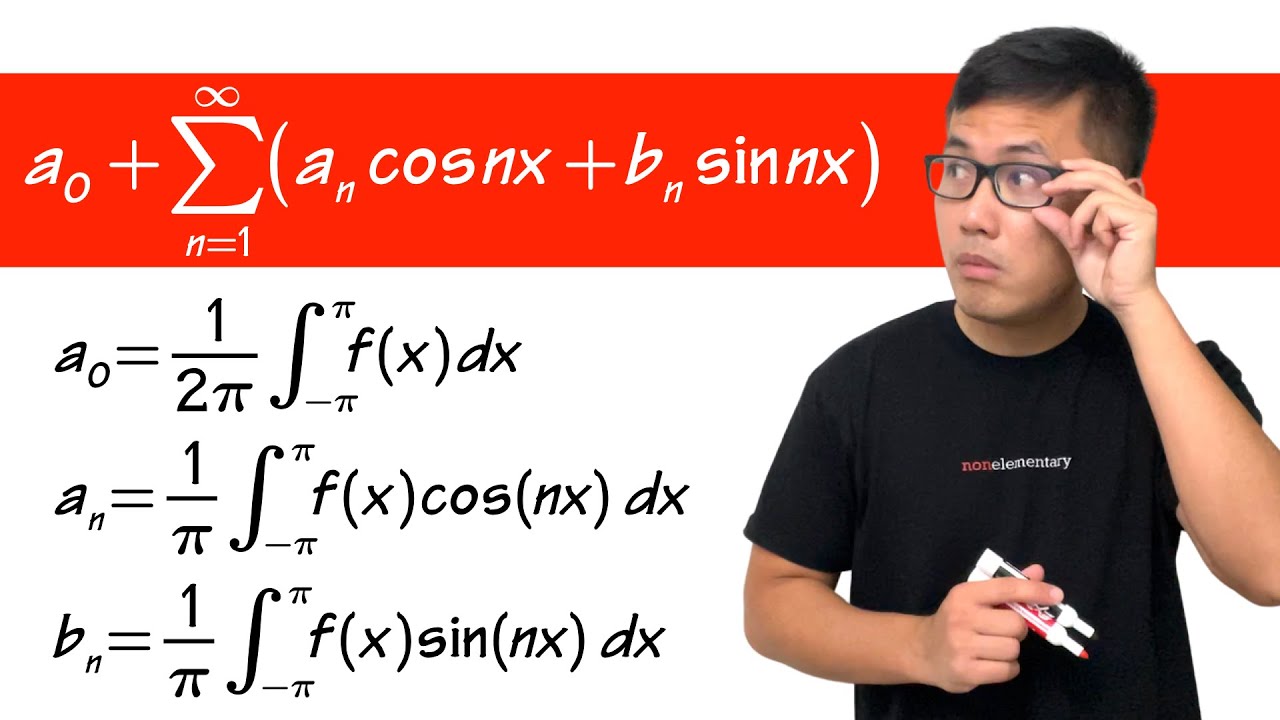Introduction and Definition of Fourier Series

Introduction

Introduction:

In mathematics and physics, Fourier series is a mathematical tool used to express a periodic function as a sum of sinusoidal functions. It was introduced by Jean-Baptiste Joseph Fourier in the early 19th century as a way to represent a complex periodic function using simpler trigonometric functions.

The concept of Fourier series is based on the idea that any periodic function can be represented as an infinite sum of sine and cosine functions of different frequencies. By decomposing a periodic function into its constituent sine and cosine waves, Fourier series allows us to analyze and manipulate periodic functions in a more intuitive and efficient manner.

Fourier series has various applications in various fields including signal processing, image compression, electrical engineering, and astronomy. It provides a powerful mathematical tool for studying and understanding the behavior of periodic systems.

In this series, we will explore the principles and applications of Fourier series, starting from the basic concepts and gradually delving into more advanced topics. We will discuss the Fourier series representation of periodic functions, the convergence of Fourier series, and the properties and applications of Fourier transforms.

By the end of this series, you will have a solid understanding of Fourier series and its applications, and be able to apply these concepts to solve problems and analyze periodic functions in various fields. Let’s get started!

Definition of Fourier Series

Fourier series is a mathematical representation of a periodic function using sine and cosine functions, or complex exponentials. It allows us to decompose a periodic function into a sum of sinusoidal components of different frequencies and amplitudes. The series is named after Jean-Baptiste Joseph Fourier, who developed the concept in the 19th century.

In simpler terms, Fourier series helps us express complex periodic functions as simpler trigonometric functions. This decomposition allows us to analyze and understand the behavior of periodic signals and functions in terms of their fundamental frequency components. Fourier series has widespread applications in fields such as signal processing, image and audio compression, telecommunications, and physics.

Mathematical Properties of Fourier Series

The Fourier series is a mathematical technique used to represent a periodic function as a combination of sine and cosine functions. It has several important properties that make it a valuable tool in mathematics:

1. Periodicity: The Fourier series assumes that the function being represented is periodic with a fixed period. This means that the function repeats itself over specific intervals.

2. Linearity: The Fourier series is a linear function, meaning that it follows the principles of linearity. This property allows for the superposition of multiple functions into a single Fourier series representation.

3. Orthogonality: The sine and cosine functions used in the Fourier series are orthogonal to each other. This means that their inner product is zero when integrating over a period, except when the functions are the same or differ by a multiple of π/2. This property simplifies the calculations involved in determining the coefficients of the Fourier series.

4. Convergence: Under certain conditions, the Fourier series converges to the original function being represented. The convergence behavior can vary based on the smoothness of the function and the magnitude of its Fourier coefficients.

5. Parseval’s Theorem: This theorem states that the total energy of a function is preserved in its Fourier series representation. It relates the squared magnitudes of the Fourier coefficients to the integral of the squared function over a period.

6. Differentiation and Integration: The Fourier series allows for the differentiation and integration of periodic functions. The derivatives and integrals of a function can be calculated by manipulating the Fourier coefficients.

These properties make the Fourier series a powerful tool in various areas of mathematics and engineering, including signal processing, harmonic analysis, and partial differential equations. They enable the decomposition, analysis, and synthesis of complex functions into simpler sine and cosine components.

Applications of Fourier Series

Fourier series is a mathematical tool used to analyze and represent periodic functions in terms of their frequency components. It has various applications in various fields. Some of the applications of Fourier series are:

1. Signal processing: Fourier series is extensively used in signal processing to analyze and manipulate signals. By decomposing a signal into its frequency components using Fourier series, one can filter out specific frequencies or modify the signal to remove noise.

2. Image analysis: Fourier series is used in image analysis to extract information about the spatial frequency content of an image. Fourier series can be used to identify specific patterns or features in an image by analyzing its frequency spectrum.

3. Communications: Fourier series plays a crucial role in communication systems. It is used in modulation techniques such as frequency modulation (FM) and amplitude modulation (AM), which rely on varying the frequency or amplitude of a carrier signal to transmit information.

4. Control systems: Fourier series is used in control systems to analyze and design controllers. It helps in understanding the frequency response and stability of control systems, allowing engineers to tune and optimize the system’s performance.

5. Speech and audio processing: Fourier series is used to analyze and process speech and audio signals. It helps in speech recognition, noise reduction, and compression techniques such as MP3 where the audio signal is decomposed into frequency components in order to reduce file size.

6. Vibrations and acoustics: Fourier series is applied in the analysis and simulation of vibrations and acoustics. It helps in understanding the harmonic content of vibrations and sound waves, enabling engineers to design structures and devices that minimize unwanted vibrations and noise.

7. Quantum mechanics: Fourier series is used in quantum mechanics to describe wave functions and the wave-particle duality of particles. It helps in understanding the behavior of particles at a microscopic level.

8. Medical imaging: Fourier series is used in medical imaging techniques such as MRI and CT scans. It helps in reconstructing images from the measured Fourier coefficients, allowing doctors to analyze internal structures and detect abnormalities.

These are just a few examples of the wide range of applications of Fourier series. It is a powerful mathematical tool that finds utility in many areas of science, engineering, and technology.

Conclusion

In conclusion, the Fourier series is a powerful mathematical tool for representing periodic functions as a sum of harmonic components. It allows us to decompose complex waveforms into simpler sinusoidal components with different frequencies and amplitudes.

The Fourier series provides a way to analyze and understand the behavior of periodic signals in terms of their harmonic content. By using the Fourier series, we can find the fundamental frequency of a periodic signal, determine the amplitudes and phases of its harmonic components, and even reconstruct the original signal from its Fourier series representation.

Furthermore, the Fourier series has applications in various fields such as signal processing, communications, image analysis, and physics. It is extensively used in areas where periodic signals need to be analyzed or synthesized.

In summary, the Fourier series is a fundamental mathematical concept that helps us understand and manipulate periodic signals effectively. Its applications are widespread, making it an essential tool in many areas of science and engineering.

Topics related to Fourier Series