Definition of Complex Conjugate and Properties of Complex Conjugates

Definition of Complex Conjugate

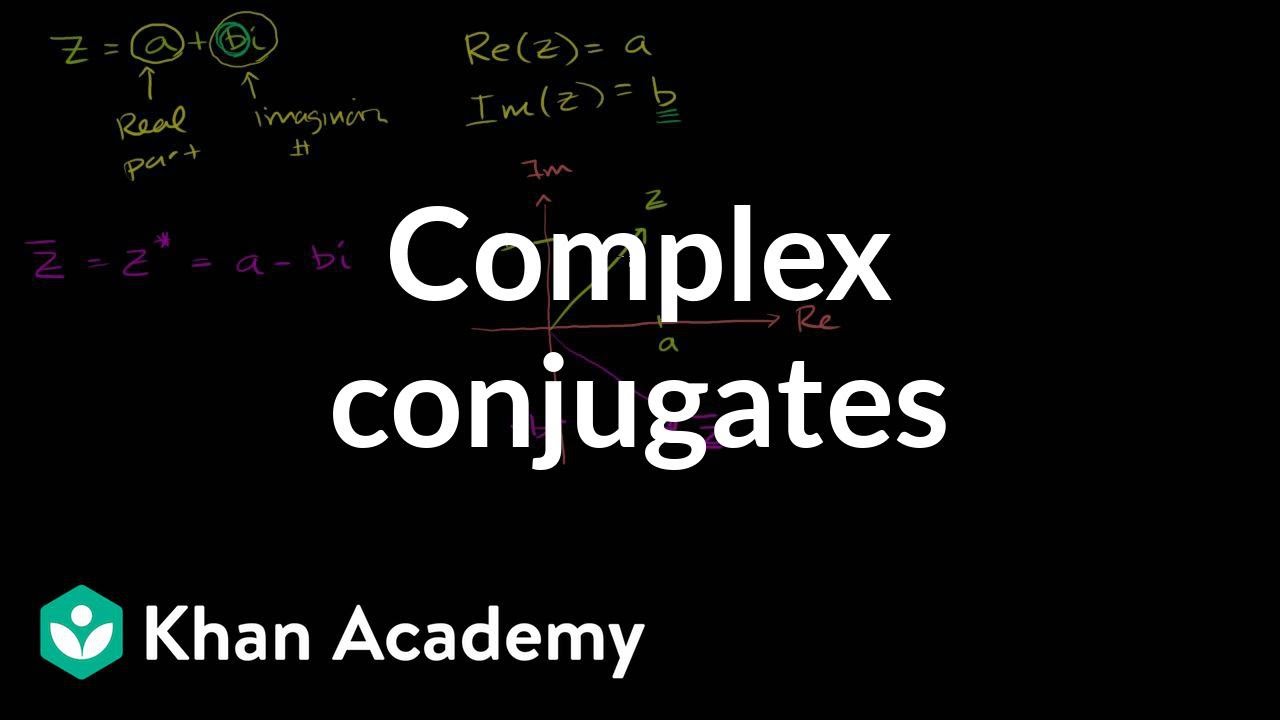
The complex conjugate of a complex number is formed by changing the sign of its imaginary part. Suppose we have a complex number z = a + bi, where a and b are real numbers and i represents the imaginary unit (√(-1)). The complex conjugate of z is denoted by z* or z bar and is given by z* = a – bi. In other words, the complex conjugate of a complex number is obtained by flipping the sign of its imaginary part.

Properties of Complex Conjugates

The complex conjugate of a complex number is a number that has the same real part but an opposite sign for the imaginary part. In other words, if we have a complex number of the form a + bi, its complex conjugate is a – bi.

Here are some properties of complex conjugates:

1. The product of a complex number and its complex conjugate is always a real number. For example, if z = a + bi, then z * z̅ = (a + bi)(a – bi) = a^2 + b^2 is a real number.

2. The sum of a complex number and its complex conjugate is always a real number. For example, if z = a + bi, then z + z̅ = a + bi + a – bi = 2a is a real number.

3. The difference of a complex number and its complex conjugate is always a purely imaginary number. For example, if z = a + bi, then z – z̅ = a + bi – a – bi = 2bi is a purely imaginary number.

4. The modulus of a complex number and its complex conjugate are equal. The modulus of a complex number z = a + bi is given by |z| = √(a^2 + b^2), and the modulus of its complex conjugate z̅ = a – bi is also |z̅| = √(a^2 + b^2).

5. The conjugate of a conjugate is the original complex number. In other words, if z = a + bi, then (z̅)̅ = a – bi = z.

These properties of complex conjugates are useful in various areas of mathematics, such as complex analysis and algebraic equations.

Operations Involving Complex Conjugates

In mathematics, the complex conjugate of a complex number is formed by changing the sign of its imaginary part.

To find the complex conjugate of a complex number, you switch the sign of the imaginary part. For example, the complex conjugate of the complex number a + bi is a – bi.

Complex conjugates are often used in operations involving complex numbers. One common operation is multiplication. When multiplying two complex numbers, you can use the property that the product of a complex number and its complex conjugate is a real number.

For example, let’s multiply (a + bi) and its complex conjugate (a – bi): (a + bi)(a – bi). Using the FOIL method, you get: a^2 – abi + abi – (bi)^2. Simplifying further, you get: a^2 – b^2(i^2). Since i^2 is equal to -1, the expression becomes a^2 + b^2.

This shows that the multiplication of a complex number and its complex conjugate results in a real number.

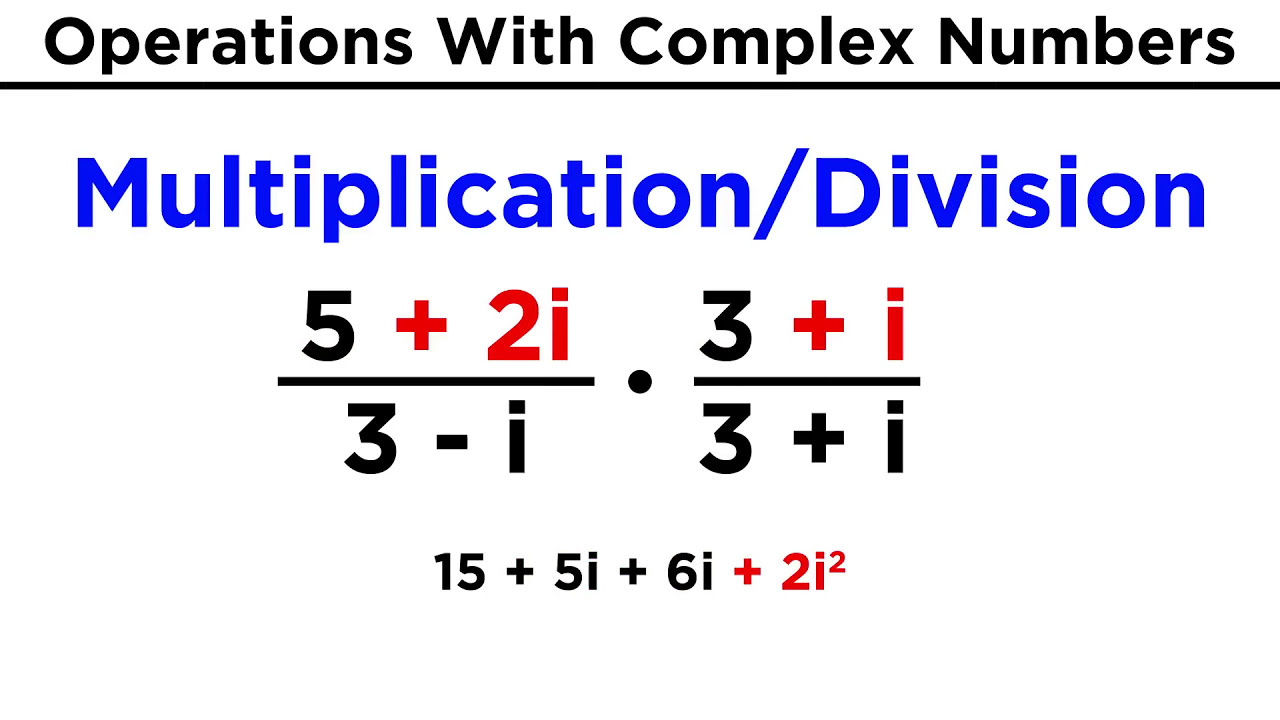
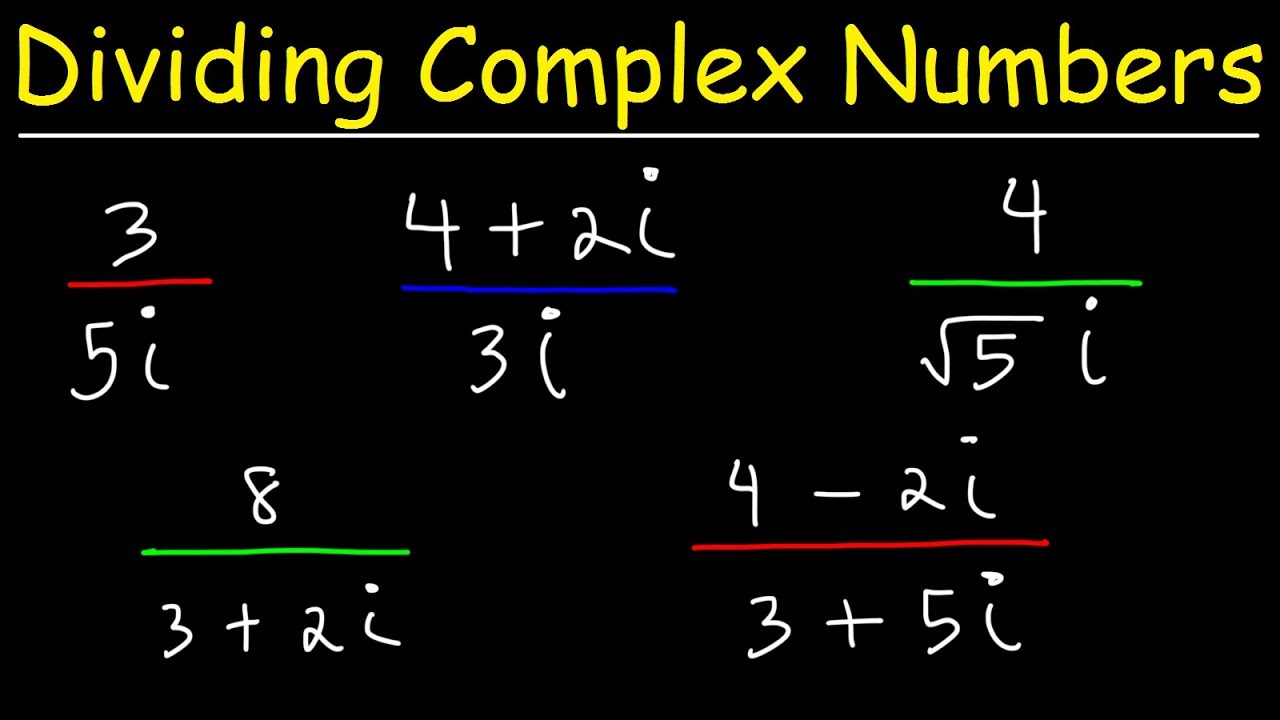
Complex conjugates are also useful for dividing complex numbers. When dividing a complex number by its complex conjugate, you again get a real number.

For example, let’s divide a + bi by its complex conjugate: (a + bi) / (a – bi). To simplify this, you can multiply both the numerator and denominator by the complex conjugate of the denominator, which is (a + bi). This gives: (a + bi)(a + bi) / (a – bi)(a + bi). Simplifying, you get: (a^2 + abi + abi + b^2i^2) / (a^2 – abi + abi – b^2i^2). Using i^2 = -1, this further simplifies to: (a^2 + b^2) / (a^2 + b^2). The result is 1, which is a real number.

In summary, the complex conjugate of a complex number is formed by changing the sign of its imaginary part. They are often used in operations involving complex numbers, such as multiplication and division, where they help simplify expressions and result in real numbers.

Applications of Complex Conjugates

Complex conjugates have a wide range of applications in various areas of mathematics and physics. Some of the key applications include:

1. Simplification of Complex Numbers: When adding or subtracting complex numbers, the complex conjugates are used to eliminate the imaginary parts and simplify the expression.

2. Finding Roots of Polynomials: The complex conjugate theorem states that if a polynomial has complex roots, they always occur in conjugate pairs. This property is used to find the remaining roots of a polynomial equation.

3. Division of Complex Numbers: To divide complex numbers, you multiply the numerator and denominator by the complex conjugate of the denominator. This process leads to simpler expressions and makes division easier.

4. Expressing Complex Numbers in Trigonometric Form: Complex conjugates are used to convert complex numbers from the rectangular form (a + bi) to the trigonometric form (r * cosθ + r * sinθ).

5. Eigenvalues and Eigenvectors: Complex conjugates play a role in linear algebra when finding eigenvalues and eigenvectors. They assist in determining complex eigenvalues of matrices.

6. Signal Processing: In signal processing, complex conjugates are often used to compute the complex conjugate of a signal. This operation is necessary for various applications, such as designing filters and modulation schemes.

7. Fourier Transform: In Fourier analysis, complex conjugates are utilized to prove important properties of the Fourier transform, such as the symmetry properties of real signals in the frequency domain.

8. Quantum Mechanics: Complex conjugates are extensively used in quantum mechanics to calculate probabilities and expected values of observables. The square of the absolute value of a complex conjugate quantity represents the probability of finding a particle in a particular state.

9. Electromagnetic Waves: In the study of electromagnetic waves, complex conjugates are employed to define the complex electric and magnetic fields. By taking the complex conjugate of these fields, one can describe the time-reversed behavior of waves.

These are just a few examples of how complex conjugates are applied across different branches of mathematics and physics. The concept of complex conjugates is fundamental and provides a valuable tool for simplification, computation, and analysis involving complex numbers and related topics.

Conclusion

In conclusion, the complex conjugate of a complex number is obtained by changing the sign of the imaginary part while keeping the real part the same. This operation is denoted by adding a bar or a prime to the original complex number. The complex conjugate is important in various mathematical applications, such as finding the magnitude of complex numbers, solving equations involving complex numbers, and performing operations like addition, subtraction, and multiplication. Additionally, the complex conjugate plays a role in the representation of complex numbers in polar form and is helpful in simplifying expressions involving complex conjugates.

Topics related to Complex conjugate