Introduction
Introduction:
Noether’s second fundamental theorem is a significant result in the field of mathematics, particularly in the study of mathematical physics and calculus of variations. This theorem, named after the German mathematician Emmy Noether, establishes a deep connection between symmetries, conservation laws, and the equations of motion.
Noether’s first fundamental theorem, which states that every differentiable symmetry of a physical system corresponds to a conservation law, laid the foundation for her second fundamental theorem. In this theorem, Noether explores the inverse relationship: how the existence of certain conservation laws can imply the presence of symmetries in a system.
Noether’s second fundamental theorem:
Noether’s second fundamental theorem encompasses a broad range of applications, but at its core, it asserts that under certain conditions, the existence of a conservation law implies the existence of a symmetry. In other words, if a physical system possesses a certain conservation law, then there must be some underlying symmetry that gives rise to it.
To understand this theorem more concretely, consider a system described by a set of differential equations. If there exists a conserved quantity, such as energy, momentum, or angular momentum, that remains constant throughout the system’s evolution, then Noether’s second fundamental theorem guarantees the existence of a corresponding symmetry.
This theorem has profound implications in various areas of physics and mathematics. It provides a powerful tool for analyzing physical systems, as it allows us to uncover hidden symmetries by observing their associated conservation laws. In turn, these symmetries can provide valuable insights into the underlying structure and behavior of the system.
Noether’s second fundamental theorem has found wide applications in fields such as classical mechanics, quantum mechanics, field theory, and general relativity. It has contributed to the development of fundamental theories, such as Einstein’s theory of gravity and the standard model of particle physics.
Overall, Noether’s second fundamental theorem is a fundamental result that sheds light on the intricate relationship between symmetries, conservation laws, and the equations of motion. It provides a powerful and elegant way to uncover symmetries in physical systems and has had a profound impact on both theoretical and applied mathematics.
Noether’s second fundamental theorem
Noether’s second fundamental theorem, also known as Noether’s second theorem, is a result in mathematical physics named after the German mathematician Emmy Noether. The theorem establishes a connection between symmetries in a physical system and conserved quantities.
In its essence, Noether’s second fundamental theorem states that if a physical system possesses a continuous symmetry, then there exists a conserved quantity associated with that symmetry. Conversely, if a conserved quantity exists in a system, there must be a corresponding symmetry.
The theorem has profound implications in the field of theoretical physics, especially in areas like classical mechanics, quantum mechanics, and field theory. It allows physicists to determine conserved quantities, such as energy, momentum, and angular momentum, by analyzing the symmetries of a system.
Noether’s second fundamental theorem is a powerful and elegant tool that has been instrumental in enhancing our understanding of the fundamental laws of nature. It provides a deeper insight into the interplay between symmetries and conservation laws, transforming the way physicists approach the study of physical systems.
Significance in mathematics
Noether’s second fundamental theorem, also known as Noether’s theorem on symmetry, is of significant importance in mathematics, particularly in the field of theoretical physics. The theorem establishes a deep connection between symmetries in a physical system and the conserved quantities associated with these symmetries.
Specifically, the theorem states that for every continuous symmetry of a physical system, there exists a corresponding conserved quantity. In other words, if a physical system remains unchanged under transformations such as translations in space, rotations, or time shifts, then there is a conserved quantity in that system.
This theorem has profound implications in various areas of physics, including classical mechanics, quantum mechanics, and field theory. It allows physicists to identify and understand the underlying principles governing the behavior of physical systems. Additionally, Noether’s second fundamental theorem provides a powerful tool for analyzing and solving problems in theoretical physics, as it allows for the identification of conserved quantities, which can simplify calculations and provide valuable insights into the dynamics of a system.
In essence, Noether’s second fundamental theorem unveils the hidden structure and organization within physical systems through the lens of symmetries. It has played a crucial role in shaping our understanding of fundamental laws and principles in physics and has paved the way for numerous advancements in the field.
Applications and examples
Noether’s second fundamental theorem, also known as Noether’s theorem of the second kind, states that every differentiable symmetry of the action principle in field theory gives rise to a conservation law.
In simple terms, if a physical system exhibits a certain symmetry or invariance, then there will be a corresponding conserved quantity associated with that symmetry.
Here are a few applications and examples of Noether’s second fundamental theorem:
1. Conservation of Energy: The invariance of physical laws under time translation symmetry leads to the conservation of energy. This means that if a system’s dynamics do not change with time, its total energy remains constant. This is a fundamental principle in various branches of physics.
2. Conservation of Angular Momentum: The rotational symmetry of physical laws leads to the conservation of angular momentum. For example, the conservation of angular momentum in planetary motion is a consequence of the rotational symmetry of the laws of gravity.
3. Conservation of Linear Momentum: The translational symmetry of physical laws gives rise to the conservation of linear momentum. This means that if the laws governing the system remain the same regardless of the position in space, the total linear momentum of the system remains constant.
4. Charge Conservation: The gauge symmetry of electromagnetic interactions gives rise to the conservation of electric charge. This means that the total electric charge within a system remains constant, and no charge can be created or destroyed.
5. Conservation of Lepton Number: The conservation laws of certain fundamental particles, such as electrons and neutrinos, arise from the underlying symmetries in particle physics. For example, the conservation of lepton number guarantees that the total number of leptons (electron-like particles) minus the total number of antileptons is constant in a particle reaction.
The examples above illustrate how Noether’s second fundamental theorem finds applications in various areas of physics, ranging from classical mechanics to quantum field theory. By identifying symmetries in physical systems, this theorem helps uncover the underlying conservation laws that govern the behavior of these systems.
Conclusion
The conclusion of Noether’s second fundamental theorem is that for any infinitesimal symmetry of the action functional, there exists a conserved quantity associated with that symmetry. This theorem is a significant result in the field of mathematics and physics, as it establishes a profound connection between symmetries and conservation laws. It has had wide-ranging implications in various areas of physics, particularly in the study of classical mechanics and field theory.
Topics related to Noetherʼs second fundamental theorem
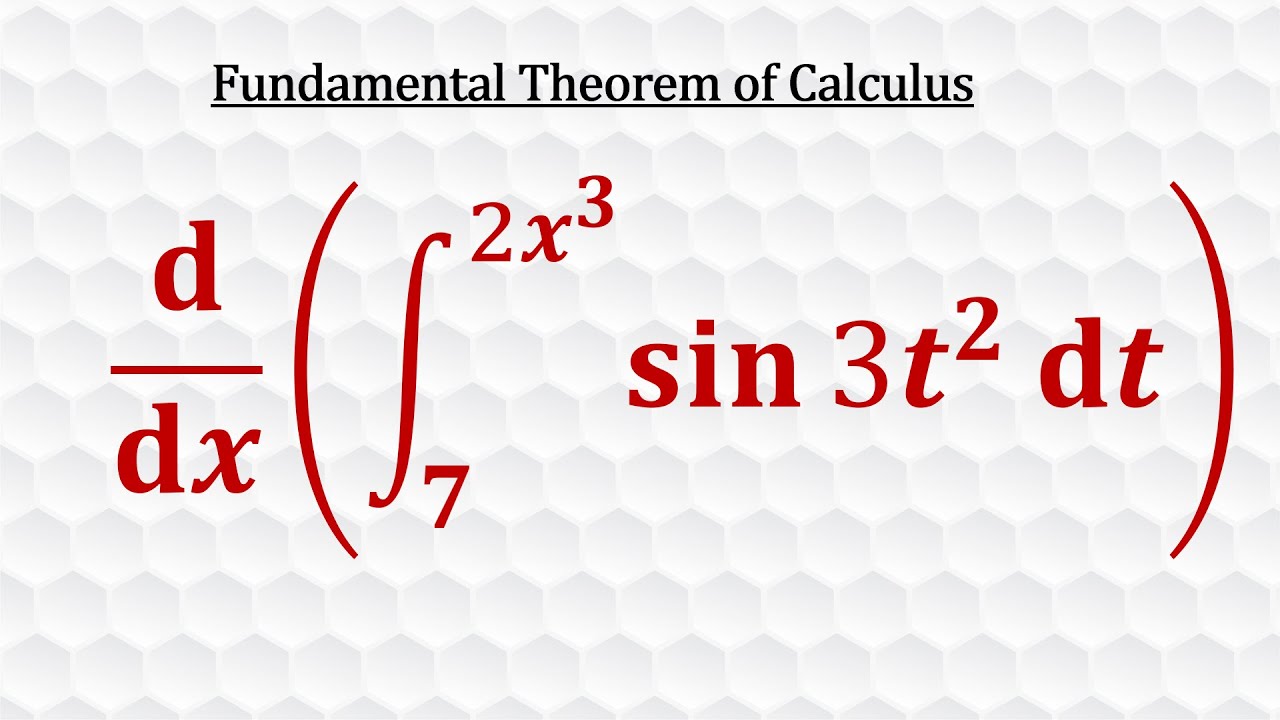
Second fundamental theorem of Calculus || A simple application ||@mathematicswithgoy – YouTube
Second fundamental theorem of Calculus || A simple application ||@mathematicswithgoy – YouTube
The Second Fundamental Theorem of Calculus – YouTube
The Second Fundamental Theorem of Calculus – YouTube
The second fundamental theorem of calculus. #math #apcalc #apcalculus #derivative #integral – YouTube
The second fundamental theorem of calculus. #math #apcalc #apcalculus #derivative #integral – YouTube
Second fundamental theorem and chain rule | MIT 18.01SC Single Variable Calculus, Fall 2010 – YouTube
Second fundamental theorem and chain rule | MIT 18.01SC Single Variable Calculus, Fall 2010 – YouTube
Fundamental Theorem of Calculus – Part I – YouTube
Fundamental Theorem of Calculus – Part I – YouTube
Fundamental Theorem of Calculus Explained | Outlier.org – YouTube
Fundamental Theorem of Calculus Explained | Outlier.org – YouTube
Fundamental theorem of algebra | Polynomial and rational functions | Algebra II | Khan Academy – YouTube
Fundamental theorem of algebra | Polynomial and rational functions | Algebra II | Khan Academy – YouTube
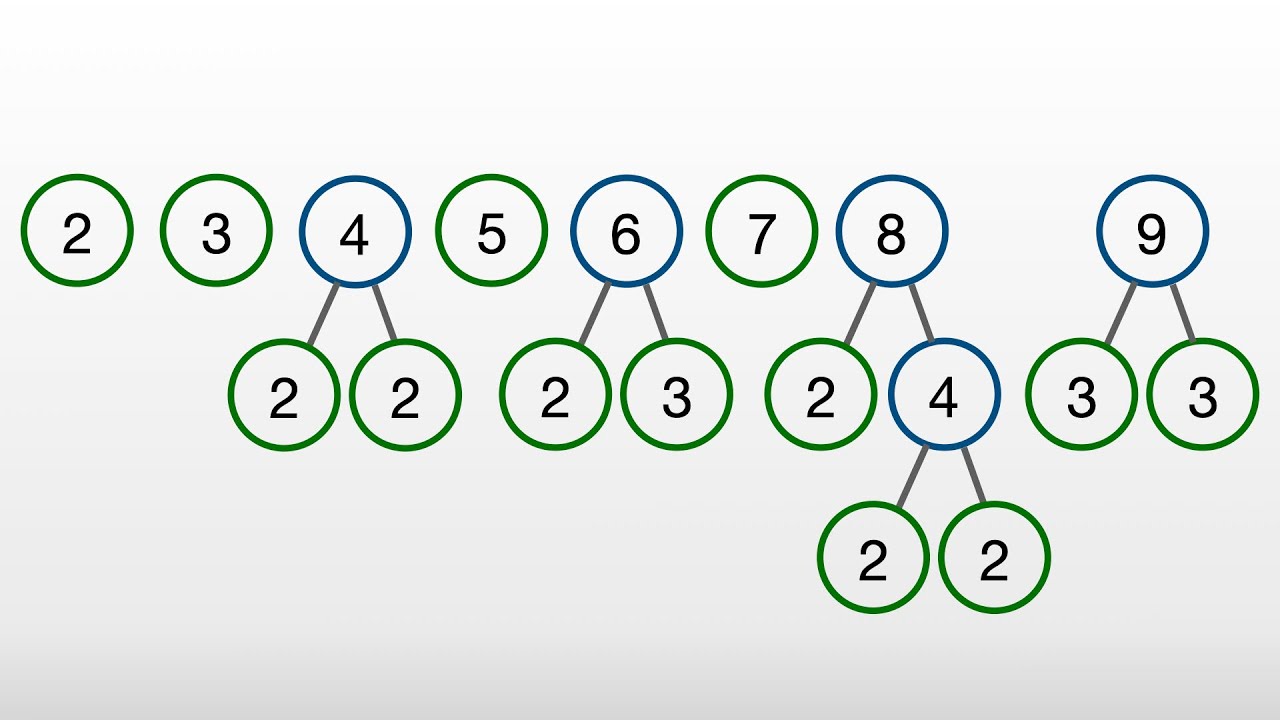
Number Theory | Fundamental Theorem of Arithmetic – YouTube
Number Theory | Fundamental Theorem of Arithmetic – YouTube
Fundamental Theorem of Calculus Part 2 – YouTube
Fundamental Theorem of Calculus Part 2 – YouTube
The Fundamental Theorem of Arithmetic – YouTube
The Fundamental Theorem of Arithmetic – YouTube

Peter Scholze is a distinguished German mathematician born on December 11, 1987. Widely recognized for his profound contributions to arithmetic algebraic geometry, Scholze gained international acclaim for his work on perfectoid spaces. This innovative work has significantly impacted the field of mathematics, particularly in the study of arithmetic geometry. He is a leading figure in the mathematical community.